Categorical Propositions

 

In logic, a categorical proposition, or categorical statement, is a proposition that can be analyzed as being about classes/ categories,  that asserts or denies that all or some of the members of one category (the subject term) are included or excluded from another (the predicate term). Such propositions affirm or deny that some class S is included or excluded from another class P, in whole or in a part.

There are four types of categorical proposition, each of which is given a vowel letter A, E, I and O.

 

Universal Affirmative Proposition:

A universal affirmative proposition says that every member of the first class is also the member of the second class.

A= ‘All S is P’.

For example:

All senators are male.

 

 

Universal Negative Proposition:

A universal negative proposition says that the first class is wholly excluded from the second class.

E= ‘No S is P’.

For Example:

No doctors are citizens.

Particular Affirmative Proposition:

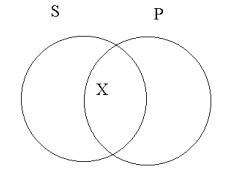
A particular affirmative proposition says that at least one member of the class S is also the part of the class P.

I= ‘Some S is P’.

For Example:

Some chocolates are expensive.

Particular Negative Proposition:

A particular negative proposition says that at least one member of the class S is excluded from whole of the class P.

O= ‘Some S is not P’.

For Example:

Some clothes are not warm

Quality:

The quality of a categorical proposition is affirmative if it affirms class membership E.g., All S is P, Some S is P. The quality of a categorical proposition is negative if it denies class membership E.g., No S is P, Some S is not P.

 

Quantity:

The quantity of a categorical proposition is universal if it makes a claim about every member of the class denoted by the subject term E.g., All S is P, No S is P. The quantity of a categorical proposition is particular if it makes a claim about just some member of the class denoted by the subject term E.g., Some S is P, Some S is not P.

 

Distribution:

A = ‘All S is P’, only ‘S’ is distributed.

E= ‘No S is P’, both ‘S’ and ‘P’ are distributed.

I= ‘Some S is P’, no distribution takes place.

O= ‘Some S is not P’, only ‘P’ is distributed.