The area around a charge in which, if a test charge introduced, will experience some force due to the charge, is called the electric field of that charge.
A charge sets up the electrical field and the second charge interacts with the electrical field of the first charge. In analogy with gravitational field, we define the electrical field E due to a charge as force per unit test charge, exerted by that charge.
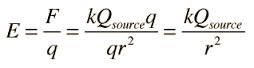
The direction of electric field is same as that of force since test charge is a positive scalar. The SI unit of electric field is newton per coulomb (N/C), although it is more often given in the units of volt per meter (V/m).
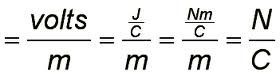
The electric field acts as the intermediary in the interaction between two charges. One charge sets the electric field in the surrounding space. This field then acts on the another charge and exerts force on it. The force that the two charges exert on each is equal and opposite if the magnitude and sign of charges is same on the two charges. If the charges are different then the electric fields may be quite different.
Now we consider the point charges. A point charge is a very small charge but it should be noted that point charge can never be smaller than the elementary charge e (= 1.602*10^-19 C)
Let a positive test charge q' be placed at a small distance r from a point charge q . Then the coulomb force F due to point charge on test charge is equal to :-
F = kqq'/r.r
And the electric field E due to point charge is :-
E = F/q'
E = kq/r.r
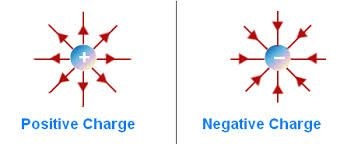
The direction of E is same as that of the direction of F, along a radial line from from point charge q. The direction is outward if the point charge is positive and is inward if the point charge is negative.
To find the electric field due to n number of point charges, we first find the electric field due to n indiviual charges. And then by adding these electric fields we can find the electric field due to n number of charges. The sum is a vector sum taken over all charges. This sum is an example of superposition principle which states that "at a given point the electric field due to separate charge distributions superimpose separately." This principle may fail when the magnitude of charges is very large.